
Chemical Kinetics
Chemical kinetics is a branch of physical chemistry that deals with the rate of chemical reactions and the mechanism through which reactants are transformed into products.
Unlike thermodynamics, chemical kinetics provides insights into the actual path taken by molecules during a chemical reaction. It explains how fast or slow a reaction proceeds and helps in understanding reaction control and mechanisms.
Rate of Reaction
The rate of reaction is defined as the change in the concentration of a reactant or product per unit time.
Mathematical expression:
Rate = Δx / Δt
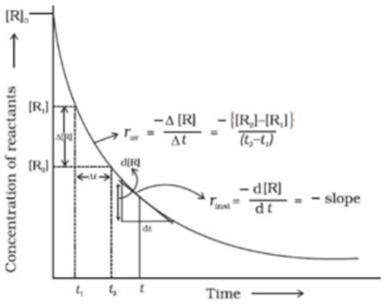
Instantaneous Rate of Reaction
The concentration-time graph of a reaction is usually curved. To calculate the rate at any instant (called the instantaneous rate), the curve is divided into infinitesimally small time intervals.
Expression:
Instantaneous Rate = dx / dt
To find the overall rate of reaction, we can sum or integrate the rate across the entire time interval:
Rate of reaction = ∫₁ⁿ (dx/dt)
- For reactants: Rate = -dx/dt (negative sign indicates decreasing concentration)
- For products: Rate = +dx/dt (positive sign indicates increasing concentration)
Unique Rate of Reaction
Unique rate is defined as the rate of change in concentration of a species divided by its stoichiometric coefficient in the balanced equation.
It is the same for all species in the reaction when adjusted for their coefficients, unlike the average rate which may differ for each.
General expression for a reaction:
aA + bB → cC + dD Unique Rate = - (1/a) × d[A]/dt = - (1/b) × d[B]/dt = + (1/c) × d[C]/dt = + (1/d) × d[D]/dt
Example
For the reaction:
N₂ + 3H₂ ⇌ 2NH₃
The rates of change for each species differ due to different stoichiometric coefficients. For instance, hydrogen reacts three times faster than nitrogen.
Unique rate expression:
Unique Rate = - (1/1) × d[N₂]/dt = - (1/3) × d[H₂]/dt = + (1/2) × d[NH₃]/dt





